
Fission Track Fundamentals (above)
Fission tracks form as Uranium spontaneously fissions into two high energy particles that barrel through the crystal lattice leaving behind a wake of damage. The tracks can be used as a proxy for a radioactive daughter product allowing us to calculate their cooling age.
When heated, the crystal lattice repairs itself causing the tracks to "anneal" or shorten. With enough heat, they disappear entirely and the apparent age will decrease.
Frictional heat will cause a localized drop in fission track age and mean track length near the fault.
Fission Track Annealing (below)
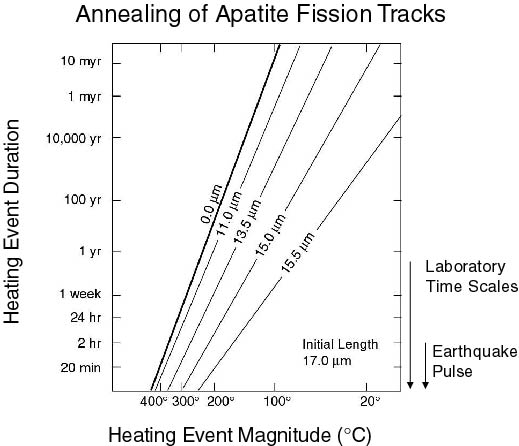
The rate of fission track annealing follows an Arrhenius law. The rate of fission track annealing is very strongly dependent on the magnitude of the temperature and less dependent on the duration, as well as crystal chemistry. In apatite, tracks completely anneal in 20 min at 400 degrees C. To completely anneal tracks at 200 degrees C, it would take nearly 40 years. The annealing laws are determined by exposing samples to different heating events and plotting them on graphs like the one shown below. By assuming a form for the annealing law, researchers can extrapolate from laboratory time scales to geologic time scales. Over time, researchers have assumed slightly different forms for the annealing relationship to provide better fits to experimental data. We use the annealing relationships of Laslett et al. (1987) and Ketcham et al. (2000).

Calculating the Thermal History at the sample locality (below)
Since all the tracks form at about the same length and they accumulate over time, we can use measurements of the length distributions to calculate the long-term thermal history of a sample. It works like this: The most recent tracks that form are only exposed to the present temperature (at the surface) where annealing is really slow. These tracks will be at the same length as when they formed. Older tracks, however, may have experienced hotter temperatures and would therefore have annealed some. The oldest tracks will have experienced the same heating as the "older" tracks mentioned a moment ago, but also experienced hot temperatures from periods before that and will be the shortest of all. By collecting a statistical distribution of the lengths of tracks, we can reconstruct a complete thermal history of the sample. To create the plot below, we perform a Monte Carlo simulation to try to create thermal histories that do a good job of fitting the original data.
Such a thermal history can give fissions-track modelers information about the timing of uplift and exhumation. We will use it to determine the depth of the samples during faulting and come up with a background thermal history to facilitate further forward modeling of fission tracks.
Unfortunately, track lengths are excruciatingly painful to measure and we can only record a certain number of lengths, leading to ambiguity in the thermal history. The "n=" in the figure above indicates 118 tracks were measured. While the best fits are fairly robust, other reasonable fits can be constructed that fit the data within the uncertainty.


Forward Modeling Fission-Track and Frictional Heat (above)
To construct these curves, we start by determining the background thermal history over geologic time of samples far from the fault (tens of meters away). We then modify that thermal history by adding short pulses of high temperatures to simulate the heating effects from individual earthquakes. We ignore the cumulative effects of heating (such as would be observed in surface heat flow) because those effects are already included in the long-term thermal history of the sample a few tens of meters from the fault (both would have nearly identical long-term thermal histories because they are very close to one another). The precise magnitude of the frictional heating event is determined by our model of heat generation and conduction and depends very strongly on distance away from the fault (with the relative magnitude of each point determined by the solution to the conduction equation). Samples close to the fault will be exposed to very high temperatures while those farther from the fault may not experience much of a temperature increase at all. The magnitude of heating also depends on the amount of fault slip, the depth of the samples (which determines the amount of normal stress), and the coefficient of friction on the fault. For the above example, we use our best estimates for these parameters (Depth: 2.3 km, Slip in earthquake: 4 m). We calculate the temperature increase for that earthquake assuming a range of possible apparent coefficients of friction (0.1 - 0.8). A point at each distance away from the fault with it's unique thermal history is plugged into a forward model of annealing to calculate the expected age and track length distribution for that point. Because the two sides of the fault have slightly different long-term thermal histories, we calculate separate curves for each side. Even though these curves are for the same simulated earthquakes, the width of the reset region is wider on the left (southwest) side of the fault because of the differences in the background thermal history.